Something cool out of microsoft?
Very neat simple “programming game”. Like Scratch, but 3d and pretty.
Its now available for PC (originally only xbox).
Devil’s Tuning Fork
Don’t know if you’ve seen this, but its a very cool student-made computer game whose premise is awesomely original. Devil’s Tuning Fork is a first-person game where you have no “vision”. The only way you can see is if you sound your tuning fork, and then you can “see” the sound waves as they bounce back to you. Echo-location like a bat. Very cool, and very impressive for a student project.
Did I mention that it’s free? (sadly only for Windows)
Bricks (WCYDWT)
How many pallets of brick,
do you need to make these,
or
this?
I’ll try this problem out for the first day of class in Applied Math. What extra information do they need?
Dynamic Geometry
Dynamic Geometry Software
I have access to Geometer’s Sketchpad at school; its installed on a bunch of laptops and in a couple of computer labs. Geogebra is a similar program but I do not have much experience with it.
For the past 4 years I’ve done various “labs” with the Geometry students where they create a set of objects in sketchpad and then measure the different characteristics. From these measurements they are supposed to write a corresponding theorem to fit the data. For instance they would create the following object: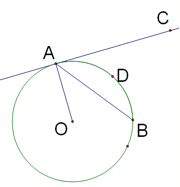 
They would then measure the angle CAO and the arc ADB to find out that the measure of the angle is exactly half that of the arc. The top quarter of students would have this written down after putting some words in their pencil: “The measure of the angle formed by a tangent and a chord is half the measure of the arc that is inside“. My “putting words in their pencil” is the following leading question: 
(the full document and its 49 steps are here: http://drop.io/circleAnglesLab )
49 steps? Are you kidding?
Am I giving the students too much help?
(or as Dan Meyer would say: “be less helpful”)
The reason there are 49 steps to the document is that this is the first time the students are seeing geometer’s sketchpad and it’s less than intuitive on how to measure an angle or an arc with this software. The document was created when I thought that it’d be best to give the kids as precise of a path as possible to the truth.
Overall I think this type of project is a good idea for how to teach some sections of geometry, but I’d like to be less focused on the steps and more focused on the material. Many students would carefully go through the steps and get to the end with the theorems written correctly, but still mess up the proper theorems on the assessments.
Where should I go from here?
Maybe I should have an intro “lesson” on how to use sketchpad to measure circles (demo how to measure an arc) and then set them free with a goal (Find the measure of an inscribed angle in relationship to an arc)
. High expectations are an excellent thing in a classroom, but am I asking the all the Oilers to be The Great One?
Last Question.
For those Sketchpad and Geogebra experts out there, would you recommend Geogebra over Sketchpad? I haven’t had enough time yet this summer to start playing with Geogebra and so I only have a very basic understanding of it. Thanks!
First 21st Century Project
So this year I’ve been given the privilege of trying out my teaching skillz in a new 21st century classroom in our building. I’m still unsure about how deep of “trouble” I am with this classroom. The classroom is being upgraded with smartboards (a normal and a small), 25 or so budget laptops, ideaPaint (whiteboard paint) on some of the walls, and some other assorted gear. As a level 2 tech-geek (out of a max level e) that is some exciting news.
This privilege comes with the qualifier that I try out some 21st century based Project Based Learning (or Product Based Learning depending on your given religious beliefs. From here on out it’ll be known as PBL). Of the three preps I have coming up next year, my Applied/Consumer classes are going to get the biggest makeover for PBL. My goal is that nearly the entire course will be PBL, but reality may be different.
Enough introduction.
I want to throw my first project for Applied Math at the figurative wall and see how it sticks. Here is the outline of the “Knockoff Project“. I got the idea about the students making a cellphone knockoff from the KIRF (keeping it real fake) posts on engadget. I suppose the most accurate feedback will be the students’ results, but please let me know what you think! I’m feel a bit “blind” in this PBL process and any and all feedback will be useful. Have I bitten off too much to chew or should I take a bigger bite?
Pumpkin – WCYDWT
This is a What Can You Do With This from around Halloween. The new at blogging thing has afforded me a chance to put some old material up.
I live in a half fancy – half normal city, Saratoga Springs NY. The fancy side of the town gives me opportunities to see some things that most people do not. For instance, there is a garish house villa (zillow puts it at $5.8 million) that decorates for holidays. 
So for Halloween, they put out an amazing spread of seasonal stuff, pumpkins, hay bales, pumpkins, and other assorted gourds. Anyway, the most interesting gourd they put out is the following:
They had a contest for the person who could guess the weight of the pumpkin, and the winner received a year membership at the local YMCA. Sitting on top of the pumpkin is a pint of chocolate milk from Stewarts (go easy now… I was thirsty and I happen to be 5 years old).
Fast forward to Applied Math class. We took a high resolution image and tried to work through how much the pumpkin would weigh. They worked out that they needed another pumpkin to make a comparison, and so from underneath my mathemagician cape, I pulled out a small pumpkin that would be easy to find the weight and volume. I have made the mistake in this class of leading them on too much, and while they enjoy the activity, I don’t think they get as much out of it.
Since this was the fall, I don’t remember the nitty-gritty details, but most of the groups had calculated that the weight was somewhere near 1000 pounds, and the real weight ended up being around 800 pounds. The different groups used different methods to find the volume by the picture, and surprisingly the most accurate weight estimates were the groups that used a sphere to model the volume. Next year I plan to make this a weeks worth of material, I’ll take more pictures, open up the competition to anyone in school (and if I’m bright I’ll put in on this blog in real time), and announce the answer online.
Course Evaluation
So this summer I have some curriculum days to spend at school, and as a break from creating a “21st century” project for Applied Math, I’m going through the course evaluation that my Geometry kids took on the last day of class. BTW, google forms totally rock for this. You quickly put together a list of questions (free response short or long, multiple choice, etc), and then you get a link to share. When the form gets filled in, the data gets put into a spreadsheet and you’re all set. Pretty neat stuff. Here is a pdf of feedback form: geometry course eval.
Anywho, this was the first time I’ve given a course evaluation (thanks to Matt for his template), and it was a little nerve wracking. Not because I think I’m a horrible teacher and the kids would ream into me, but because I wasn’t sure what to do with the feedback. Sort of a fear of a vacuum thing.
First topic under the gun:
Homework
On the whole the geometry students found the homework to be easy-ish and fairly graded. I graded only on completion (4pts compete, 2pts half done), and if it wasn’t done they had a chance to bring it in next class for half-credit. The vast majority of students didn’t take advantage of this, but the ones who did were grateful. There were many comments about how in the long run homework helped, and when they slipped up on homework they slipped up on tests and quizzes. I plan on using this student support for homework (!) on future classes, maybe they’ll listen a bit more when it comes from different sources.
In the long run I plan to greatly reduce the amount of points and time that homework consumes, but philosophically I feel I need to phase that in slowly with the students (Cornally-Hulk angry?) .
What did we do too much of?
I submit a wordle cloud to explain their responses: 
Is that clear then? We need (?) to spend about 18% of our time on proofs in our Geometry Regents class, so that our state can weigh proofs at about 6% of the final exam (</whining>). Not to mention that our time with proofs doesn’t get many of them very far at all. Its a frustrating topic for a lot of kids and man are they “squeaky wheels” about not getting it. The cool thing is that the quarter of the kids that do “get it” are not typically they kids who did well in previous algebra and geometry topics… I like that.
What did we do too little of?
Alright wordle time again:
Well Mr. Holmes what do you make of that? Now we did too little proofs? I think it boils down to a matter of preference, some feel like we spent too much time on Proofs because they didn’t get them, and some feel like we spent too little time on Proofs because they didn’t get them.
Interestingly enough the two topics we finished with, circles and constructions, were big too. Is that just a bias about what topics they know we did and what immediately came to mind when they filled out the form?
Overall I found this exercise to be interesting, but the muddy results screw with my logical brain. I want the results to be clear and obvious but naturally that is not going to be the case. I might get where to buy generic cialis UK more concrete answers when we get back the item analysis for our exam. I will definitely do this next year, with more one-word answers or multiple choice. The comments are great to read, but difficult to parse. I’ll finish up with a pie chart (created by google) for the difficulty of this course… I think it looks good, but who knows?
p.s. Clearly my students have awful spelling! Oh well, I’m no english teacher 😉
Robocode & Math
We’ve used a open-source tool called robocode. Basically it is a game where you program robots in Java (or .Net). The robots battle in real-time and on-screen. It was a closing project (last 4 weeks) to our Intro to Programming class, and it was excellent. They really got into battling each other’s robots and creating code to do better and better. We had a round-robin regular season, and then a double-elimination playoff bracket to see whose robot was the best. Awesome stuff, and my bot was in the bottom half!
Anyway, I promised math, and I’ll deliver:
double absoluteBearing = getHeadingRadians() + e.getBearingRadians();
double enemyX = getX() + e.getDistance() * Math.sin(absoluteBearing);
double enemyY = getY() + e.getDistance() * Math.cos(absoluteBearing);
Now don’t be scared non-CS people. Essentially what they are doing is they are finding out the position of the enemy robot by only knowing in what cardinal direction they are coming from, and knowing their (x,y) position.
Now even though I’m a math teacher, I promise, I didn’t lead them to sine and cosine! I refused to provide any real useful hints, I just plotted two points on a coordinate plane and they figured it out. I had a couple of others use the Pythagorean theorem to find the distance to a corner. One student ended up using the law of cosines to calculate the length of a side of a non-right triangle.
Good stuff, just thought you algebra II teachers would enjoy the use of those formulas in situation outside the math classroom.
Standards Based Grading
Why change?
The answer to this question for me stems from the idea that I don’t really care when the learning happens, only if the learning happens.
Part of me also sees how sbg could encourage some bit of student responsibility in their learning. They would know that if they didn’t get the stuff for a quiz, they’d have the ability to patch the holes in their knowledge.
My 3 sticking points for SBG (and solutions?):
-
Midterms/Finals
One of the most important steps for me in grasping sbg is the idea that the rare comprehensive exam can still happen: -
Memorization vs. Real Knowledge
This year I did a “trial” run of the sbg in an Algebra class for the second half of the year. This was the first time I’ve taught Algebra Regents (the Regents exam is NY’s state exam). I felt over my head in the vast number of different topics that we were required to teach. I had a difficult time keeping in my head what we’ve done and what we have coming up because many of the topics are essentially review for the kids, while other topics are entirely new to them.
Anywho, the biggest problem I had from the sbg system was when the students came after school for remediation. I hadn’t laid down many specific rules, and so they wanted my help to study and then immediately take a re-assessment for credit. They also didn’t clearly understand the boundaries between the study part (I can help) and the quiz part (I can’t). They would continually think they had a topic, sit down for the quiz, and get stumped because they had never really had it down. It all clicked when I read the following:The best modification this year was: require that if you are staying after school with me, you are either there to get help, or you are there to re-test. Never both. If you want my help, great, but you have to come back to re-test. Retesting is a no kidding, materials put away, sitting at a desk by yourself with a pencil and a calculator situation. It was a good change because: they are more likely to at least try to do some preparation on their own, and their grade is a better reflection of what they’ve learned as opposed to what they just stored in their short term memory.
Kate Nowak – Get Your Hot Fresh SBG ChecklistsDing ding ding! Ok that clicks.
-
The grades can go down?
The problem I’m having trouble processing is the idea that maybe their grades can go down too. Say a student gets a 2 out of 5 originally on a quiz, stays after school and improves all the way to a 5/5. Then a month later I retest that standard and they go down to a 3. If the students figure this out, why would they stay after school to fix the original problem if they knew that they would be retested on it later?
When kids know they’re responsible for retaining, and — this is key — they know what they’re responsible for retaining, studying becomes a guided missile of academic awesome. This is often the trouble with high school kids.
Shawn Cornally – Standards-Based Grading: Lowering Grades?I think the best of my kids can be responsible for the retention, but can the average kid do so? Is this a factor that Shawn has older and more capable kids in his classroom (physics and calculus kids)? I’m currently stumped on this issue.
Less frequent Summative Assessments (Tests): I now am free to create true ‘Midterms’ and ‘Finals.’ These tests are now more trustworthy, because students have been informed all semester about the true status of their progress. These tests now represent a chance for a student to do many things that do not have to do with specific content standards: They can practice the skill of studying as needed for college, they can practice self-assessment, and they can develop a healthy confidence for an exam that they know can’t be reattempted.
Shawn Cornally – Standards-Based Grading: Math
Since I am sending these kids to HS teachers and college professors that are going to give these types of exams, if I didn’t give these types of assessments to the kids I would feel as I’ve done a disservice.
That said, I’ll certainly be trying out sbg in all my classes next year.
Quibids.com Auction Ripoff
My dad sent me a link to quibids.com, an online auction site that allows you to buy items by bidding on them. Sounds familiar? The twist is that this site is a type of penny auction site where each bid costs money. A second catch is the auction has no set time limit. It has a count-down timer but it adds on 10 seconds each time someone bids. It’s great to see a live auction because I always imagine the disappointment had by the winning bidder as the time goes 4…3…2…1…………….14….13 (with a new winning bidder).
Anyway, this place makes a load of cash on the simplest of items: gift cards. Here is a completed auction for a $50 Outback Gift Card.
This deceptive webpage puts the savings at $18.10 for a $50 dollar card. Inconceivable!
The Consumer math kids broke down the cost to “saltyfish13”. Saltyfish13 made 43 bids and eventually paid $6.10 for the card. A hidden fact regarding the bids, is that while the bids go up by 2 cents at a time, we assume that they must cost 2 cents. However each bid is 60 cents! So saltyfish paid $25.80 for the bids and $6.10 for the card, $31.90 overall.
How does this company make money if they are giving money away on each bid?? Well even the losers to the auction pay 60 cents per bid. Since this auction went up by 2 cents at a time, there were 305 bids. The revenue for this bid is $183 plus the $6.10 for a total of $189.10 on a $50 gift card!! Wow that’s a nice profit!
We went on to talk about how there are plenty of auctions where they may lose a bit of money, but how that is certainly outweighed by the massive profits of a couple of random auctions. They picked up on the idea that the person feeling the worst must be zane51, the second-to-last person to bid on the card. Who knows how many times this person has bid to try to win this $50 card.
The students went online in pairs to find 3 types of auctions, one big profit, one break even, and one loss.
I hope to continue these type of lessons to give the students a shot at not being ripped off nearly as much as many.
My favorite part of the day was when somebody described it as a game of musical chairs, where everyone pays a fee for each round that they stay in and the final person keeps the prize. I couldn’t have (and didn’t) described it better.